Exponential - Natural G&D

The questions on this page focus on:

Growth - direct statement;
Growth - statement of the derivative;
Decay - direct statement;
Decay - statement of the derivative.

Growth	Direct statement	1. P = 1000 e^0.04t. (i) the number of bacteria (to the nearest 10) when t = 10. (ii) the rate at which the colony is increasing when t = 10. (iii) how many minutes it takes for the colony to double in size. Note: In questions asking doubling in size, halving in number, etc you can just use 2 or 0.5 etc rather than writing the new number and then dividing. Some questions do not give you numbers anyway - see question 11 below.
		2. N(t) = 1200 e^kt where t is the number of years and k is a constant. At the beginning of 2019, the population had grown to 1,500 people. (i) Show that k = 0.0558 (correct to 4 decimal places). Note t = 4 years. (ii) Find the time it will take for the population of the town to increase to 2,000. (iii) At what rate was the town growing in that year compared to the rate in 2015?
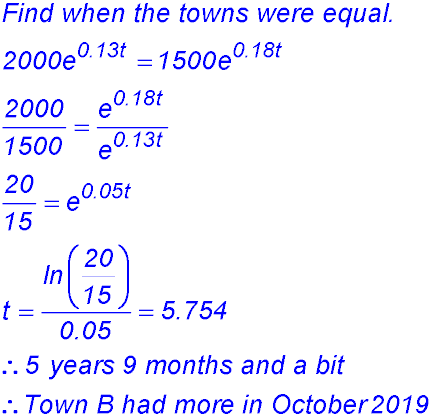
		3. The respective populations (ii) M₀ = 100 k = 1.15 × 10^-4. (iii) mass is about 63 grams. (iv) 26,000 years.N years after 1 January 2014 can be expressed as Town A: N_A = 2000 e^0.13t Town B: N_B = 1500 e^0.18t (i) the population of town A at the start of 2019. Note t = 5. (ii) Find, in which year, town B's population doubled. (iii) In which year and month did town B's population pass town A's population?
		4. D = 80e^kt (i) Show that where k is a constant. (ii) If k = 0.0018, how long (to the nearest year) will it take for the diameter of the tree to measure 90 cm.
		5. (i) Determine the values of R₀ and k (to 4 significant figures). R₀ is the number at the first count - so 200. (ii) Calculate the number of rabbits in the reserve after 20 weeks (to the nearest 10). Pests!!!! (iii) How long will it take for the number of rabbits to reach 6,000? (iv) How many rabbits per week were being added to the population after 20 weeks? Remember the rate of increase is simply k times the number of rabbits present at that time. Number added in week 20 = 0.1577 × 4,690 = 740 per week to the nearest whole rabbit That was enough - those left had to learn to share the bush!!
		6.
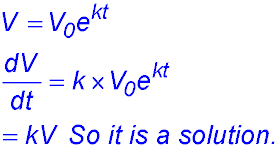
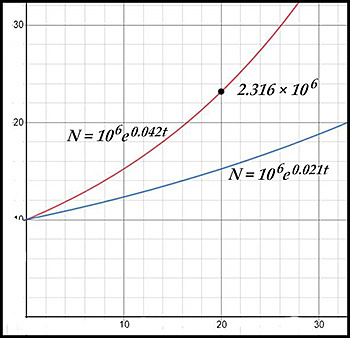
	Statement of derivative	7. (i) (ii) (iii) (iv) No - selling the property in 5 years time at $290,000 would be a long way under its projected value then. (v) If he rejected the "5 years into the future" offer and, in 8 years time from now, the value of his property was actually $595,000, then the model is not predicting the values very well. The prediction is about $667,067 which is a long way above the actual property values. It appears the value of k has reduced over the time period.
		8. (i) Bacteria are present after 20 hours: (iii) the percentage rate of increase of the population is 4.2% of the number in the culture at that time. (iv) The new equation for the number of bacteria is: N = 10⁶ × e ^0.021t (v)
		9.
		10. (i) The rate equation is 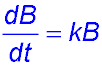. (ii) To find a value for the growth constant k. (iii) Number of bacteria on the cheese after 2 hours: (iv) At what rate were they increasing after 2 hours? Rate of increase = 0.1823 × 1440 = 162 bacteria/hour. (v) Maximum time for the cheese to be left out (with a 10% buffer on the count): Three times is 3000 bacteria but, with the safe margin, this reduces to 3,000 × 90% = 2700.
Decay	Direct statement	11. (i) To find the value of k. (ii) Number of years for 20% of the initial quantity to remain:
		12. The population in a country mining town was recorded at the start of 2010. The population P - t years later - is described by the exponential equation P = 120,000 e^-0.05t. (i) What was the initial population of the town at the start of 2010? (ii) Find the time, in years and months, it will take for the population to halve. (iii) At what rate is the population changing at the start of 2019?
		13. The mass M grams of a radioactive isotope of carbon found in a rock sample at time t is described by the equation M = M₀ e^-kt where M₀ and k are constants. (i) Prove that the rate of decay of the mass of the carbon isotope is proportional to the mass present at any time t. (ii) If there is initially 100 grams of the isotope and this mass decays to 75 grams in 2,500 years, find the values of M₀ and k. Give your value of k correct to 3 significant figures. (iii) Find the amount of the isotope present at the end of 4,000 years. Express your answer to the nearest gram. (iv) Find the time required for the mass of the isotope to decay to 5 grams. Express your answer correct to the nearest 100 years. Answer.
		14. Ten kilograms of sugar are placed in a container of water and begin to dissolve. After t hours, the amount of undissolved sugar is given by (i) Calculate the value of k given that A = 3.2 when t = 4. (ii) After how many hours does 1 kg of sugar remain undissolved?
		15. (i) (ii)
	Statement of the derivative.	16. (i) 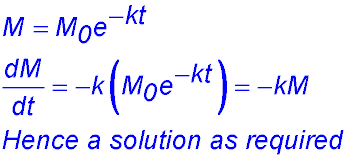(ii) (iii) .
		17. (i) (ii) Calculate the velocity and acceleration when t = 5:
		18.
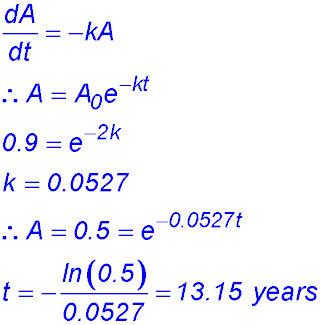		19. It the negative acceleration is proportional to its velocity - so 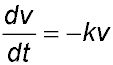. (i) . (ii) (iii) Find how long it takes for the velocity to fall to 100 m/sec (to the nearest m/sec).
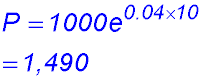
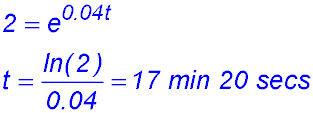
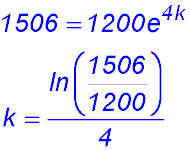
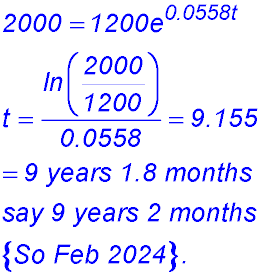
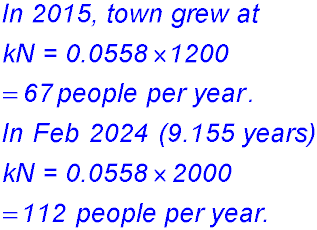
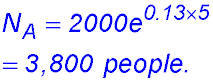
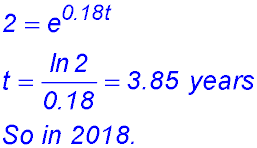
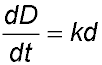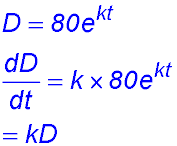
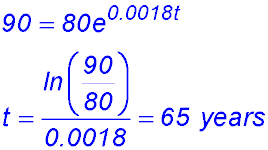
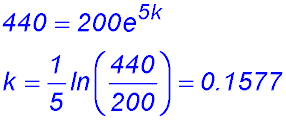
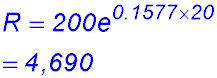
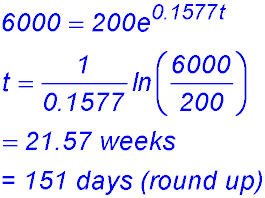
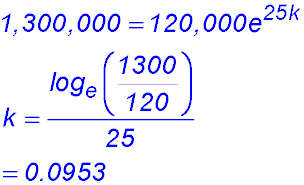
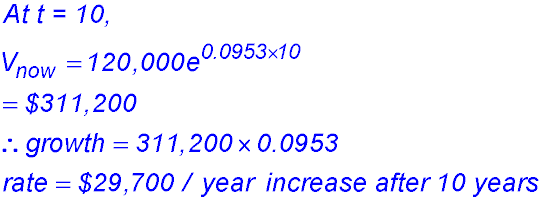
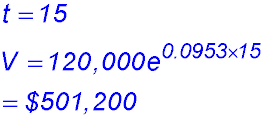
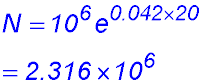
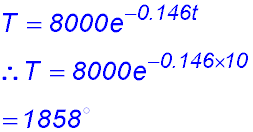
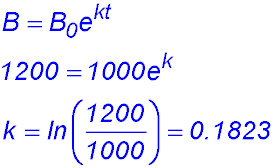
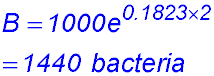
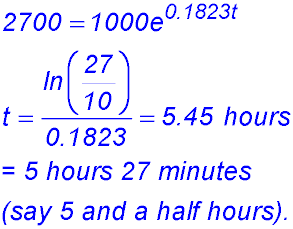
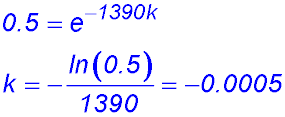
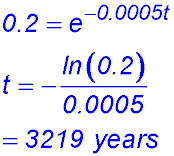
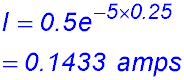
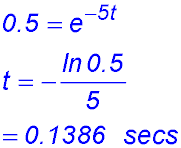
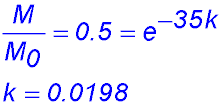
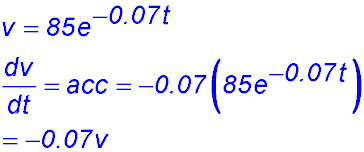
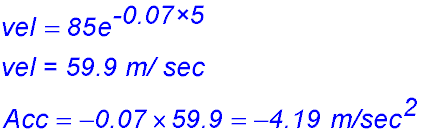
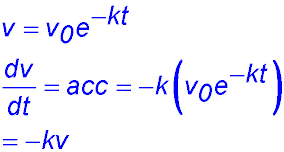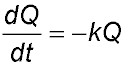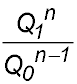		20. A quantity Q of a radioactive substance decays at a rate that at any time t is proportional to the amount remaining at that time. That is where k is a positive constant. Q₀ is the amount of the substance at t = 0. At t = T, the quantity of the substance has deceased to Q₁. (i) Show that Q = Q₀e^-kt is a solution of the differential equation . (ii) Express the value of the constant k in terms of T, Q₀ and Q₁. (iii) Show that the amount of the radioactive substance remaining at time nT can be expressed as .